Question 5.16:
Two masses 8 kg and 12 kg are connected at the two ends of a light inextensible string that goes over a frictionless pulley. Find the acceleration of the masses and the tension in the string when the masses are released.
The given system of two masses and a pulley can be represented as shown in the following figure:
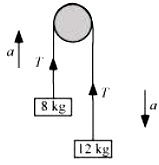
Smaller mass, m1 = 8 kg
Larger mass, m2= 12 kg
Tension in the string = T
Mass m2, owing to its weight, moves downward with acceleration a, and mass m1 moves upward.
Applying Newton’s second law of motion to the system of each mass:
For massm1 : The equation of motion can be written as:
T – m1g = ma …………… (i)
For mass m2: The equation of motion can be written as:
m2g-T=m2a ...........(ii)
Adding equations (i) and (ii), we get:
(m2 - m1)g = (m1 + m2)a
⇒a = (m2 = m1m1 + m2)g
= (12 - 812 + 8) × 10 = 2 m/s2
Therefore, the acceleration of the masses is 2 m/s2.
Substituting the value of a in equation (ii), we get:
m2g - T = m2(m2 - m1m1 + m2)g
T = (m2 - m22 - m1m2m1 + m2)g
= (2m1m2m1 + m2)g
= (2 × 12 × 812 + 8) × 10
= 2 × 12 × 820 × 10 = 96 N
Therefore, the tension in the string is 96 N.

© 2025 GoodEd Technologies Pvt. Ltd.
