The bob A of a pendulum released from horizontal to the vertical hits another bob B of the same mass at rest on a table as shown in the figure.
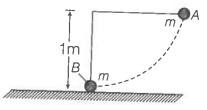
If the length of the pendulum is 1m, calculate
(a) the height to which bob A will rise after the collision.
(b) the speed with which bob B starts moving.
Neglect the size of the bobs and assume the collision to be elastic.
When ball A reaches the bottom point its velocity is horizontal hene, we can apply conservation of linear momentum in the horizontal direction.
(a) Two balls have the same mass and collision between them is elastic, therefore, ball A transfers its entire linear momentum to ball B. Hence, ball A will come to rest after the collision and does not rise at all.
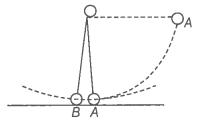
Step 2: Find speed of ball B just after the collision.
(b)Using linear momentum
Linear momentum of bob B just after collision = Linear momentum of bob A just before the collision.
As here masses of both balls are same
So,
Speed with which bob B starts moving
© 2024 GoodEd Technologies Pvt. Ltd.
