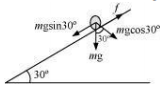
Question 7. 31. A cylinder of mass 10 kg and radius 15 cm is rolling perfectly on a plane of inclination 30°. The coefficient of static friction μs = 0.25.
(a) How much is the force of friction acting on the cylinder?
(b) What is the work done against friction during rolling?
(c) If the inclination O of the plane is increased, at what value of 8
does the cylinder begin to skid, and not roll perfectly?

© 2025 GoodEd Technologies Pvt. Ltd.
