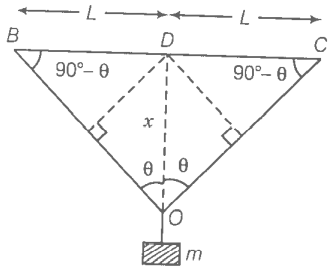
A mild steel wire of length 2L and cross-sectional area A is stretched, well within the elastic limit, horizontally between two pillars (figure). A mass m is suspended from the mid-point of the wire. Strain in the wire is:
| 1. | x22L2 | 2. | x L |
| 3. | x2L | 4. | x22L |

© 2025 GoodEd Technologies Pvt. Ltd.

