10.31 (a) It is known that density ρ of air decreases with height y as:
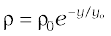
where ρ0 = 1.25 kg m–3 is the density at sea level and y0 is a constant. This density variation is called the law of atmospheres. Obtain this law assuming that the temperature of the atmosphere remains a constant (isothermal conditions). Also, assume that the value of g remains constant.
(b) A large He balloon of volume 1425 m3 is used to lift a payload of 400 kg. Assume that the balloon maintains constant radius as it rises. How high does it rise?
[Take y0 = 8000 m and ρHe= 0.18 kg m–3].
(a)
Consider a layer of thickness dy at the height y and cross-section area A.
Mass of the layer = Mass of the atoms in the layer
M=ρAdy=No. of atoms per unit volume×volume×mass of an atom
⇒ρAdy=mNAdy
⇒ρ=mN
Pressure force=weight of the layer
PA-(P+dP)A=mNAdyg
dP=-mNdyg=-ρdyg
From ideal gas equation: P=NkT=ρkTm
dP=kTmdρ
So, kTmdρ=-ρgdy
dρρ=-mgkTdy=-cdy
Integrating it:
∫ρρodρρ=-mgkTdy=-∫y0cdy
ln(ρ)-ln(ρ0)=-cy
ln(ρρ0)=-cy
ρρ0=e-cy
When y=y0⇒ρ=ρ0⇒c=1y0
⇒ρρ0=e-yy0⇒ρ=ρ0e-yy0
(b)
Density, ρ=MassVolume
=Mass of the payload+Mass of heliumVolume
=m+VρHeV
=400+1425×0.181425
ρ=ρ0e-y/y0
logeρρ0=-yy0
∴y=-8000×loge0.461.25
=-8000×-1
=8000 m=8 km

© 2025 GoodEd Technologies Pvt. Ltd.
