Ncert Exercises & Solutions

Use (i) the Ampere's law for H and (ii) continuity of lines of B, to conclude that inside a bar magnet, (a) lines of H run from the N - pole to S - pole, while (b) lines of B must run from the S-pole to N - pole.

Hint: Use the ampere-circuital law.

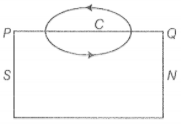
Step 1: Consider a magnetic field line of B through the bar magnet as given in the figure below.

The magnetic field line of B through the bar magnet must be a closed-loop.

Let C be the amperian loop. Then,

$\int_{Q}^{P} H \cdot dl = \int_{Q}^{P} \frac{B}{m_{0}} dl$

We know that the angle between B and dl is less than 90

$°$ inside the bar magnet. So, it is positive.

i.e.

$\int_{Q}^{P} H \cdot dl = \int_{Q}^{P} \frac{B}{μ_{0}} \cdot dl > 0$

Hence, the lines of B must run from the south pole (S) to the north pole (N) inside the bar magnet.

Step 2: According to Ampere's law,

$∴ \oint_{PQP} H . dl = 0$

$∴ \oint_{PQP} H . dl = \oint_{P}^{Q} H . dl + \oint_{Q}^{P} H . dl = 0$

As,

$\int_{P}^{Q} H \cdot dl > 0, and, \int_{Q}^{P} H \cdot dl < 0$ (i.e., negative)

It will be so if the angle between H and dl is more than 90

$°$ , so that cos

$θ$ is negative. It means the line of H must run from N-pole to S-pole inside the bar magnet.

NEET Information

courses

Company

Botany Questions

Chemistry Questions

Physics Questions

Zoology Questions