Ncert Exercises & Solutions

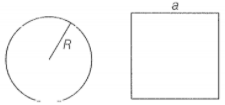
There are two current-carrying planar coil made each from identical wires of length L. $C_{1}$ is circular (radius R) and $C_{2}$ is square (side a). They are so constructed, that they have the same frequency of oscillation when they are placed in the same uniform B and carry the same current. Find a in terms of R.

Hint: Use the formula of frequency of oscillation of a bar magnet.

Step 1:

$C_{1}$ = circular coil of radius R, length L,

number of turns per unit length,

$n_{1} = \frac{L}{2 πR}$

$C_{2}$ = square of side a and perimeter L, number of turns per unit length,

$n_{2} = \frac{L}{4 a}$

$\begin{array}{l} Magnetic moment of C_{1} \\ \Rightarrow m_{1} = n_{1} {IA}_{1} \\ Magnetic moment of C_{2} \end{array}$

$\Rightarrow m_{2} = n_{2} {IA}_{2}$

$m_{1} = \frac{L \cdot I \cdot {πR}^{2}}{2 πR}$

$m_{2} = \frac{L}{4 a} \cdot I \cdot a^{2}$

$m_{1} = \frac{LIR}{2} . . . (i)$

$m_{2} = \frac{LIa}{4} . . . (ii)$

Step 2:

$\begin{array}{l} Moment of inertia of C_{1} \Rightarrow I_{1} = \frac{{MR}^{2}}{2} . . . (iii) \\ Momont of inertia of C_{2} \to I_{2} = \frac{{Ma}^{2}}{12} . . . (iv) \\ Frequrncy of C_{1} \Rightarrow f_{1} = \frac{1}{2 π} \sqrt{\frac{m_{1} B}{I_{1}}} \end{array}$

$\begin{array}{l} Frequency of C_{2} \to f_{2} = \frac{1}{2 π} \sqrt{\frac{m_{2} B}{I_{2}}} \\ According to question, f_{1} = f_{2} \end{array}$

$\begin{array}{l} \frac{1}{2 π} \sqrt{\frac{m_{1} B}{I_{1}}} = \frac{1}{2 π} \sqrt{\frac{m_{2} B}{I_{2}}} \\ \frac{I_{1}}{m_{1}} = \frac{I_{2}}{m_{2}} or \frac{m_{2}}{m_{1}} = \frac{I_{2}}{I_{1}} \end{array}$

Step 3:

Plugging the values by Eqs. (i), (ii), (iii) and (iv);

$\begin{array}{l} \frac{LIa \cdot 2}{4 \times LIR} = \frac{{Ma}^{2} \cdot 2}{12 \cdot {MR}^{2}} \\ \frac{a}{2 R} = \frac{a^{2}}{6 R^{2}} \\ 3 R = a \end{array}$

Thus, the value of a is 3R.

NEET Information

courses

Company

Botany Questions

Chemistry Questions

Physics Questions

Zoology Questions