Ncert Exercises & Solutions

Consider the L-C-R circuit shown in the figure. Find the net current i and the phase of i. Show that $i = \frac{V}{Z}$ $i = \frac{V}{Z}$ . Find the impedance Z for this circuit.

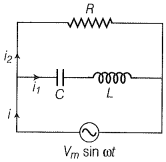
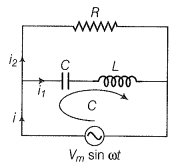
Hint: The net current will be equal to the sum of currents flowing in the two branches.

Step 1: In the given figure i is the total current from the source. It is divided into two parts

i_{2}

$i_{2}$ through R and

i_{1}

$i_{1}$ through series combination of C and L.

So, we can write; i=

i_{1}

$i_{1}$ +

i_{2}

$i_{2}$

As, V_{m} sinωt = {Ri}_{1} [from the circuit diagram]

$As, V_{m} sinωt = {Ri}_{1} [from the circuit diagram]$

\Rightarrow i_{1} = \frac{V_{m} sinωt}{R} . . . (i)

$\Rightarrow i_{1} = \frac{V_{m} sinωt}{R} . . . (i)$

Step 2: If

q_{2}

$q_{2}$ is the charge on the capacitor at any time t, then for a series combination of C and L, applying KVL in the lower Circuit as shown;

\frac{q_{2}}{C} + \frac{L d i_{2}}{d t} - V_{m} sin ω t = 0

$\frac{q_{2}}{C} + \frac{L d i_{2}}{d t} - V_{m} \sin ω t = 0$

$\Rightarrow \frac{q_{2}}{C} + \frac{L d^{2} q_{2}}{d t^{2}} = V_{m} \sin ω t [∵ i_{2} = \frac{d q_{2}}{d t}] . . . (i i)$

$L e t, q_{2} = q_{m} \sin (ω t + ϕ)$

$∴ \frac{d q_{2}}{d t} = q_{m} ω \cos (ω t + ϕ)$

$\Rightarrow \frac{d^{2} q_{2}}{d t^{2}} = - q_{m} ω^{2} \sin (ω t + ϕ)$

Step 3: Now putting these values in Eq. (ii), we get;

$q_{m} [\frac{1}{C} + L (- ω^{2})] \sin (ω t + ϕ) = V_{m} \sin ω t$

$I f ϕ = 0 a n d (\frac{1}{C} - L ω^{2}) > 0,$

$t h e n q_{m} = \frac{V_{m}}{(\frac{1}{C} - L ω^{2})} . . . (i v)$

$F r o m E q . (i i i), i_{2} = \frac{d q_{2}}{d t} = ω q_{m} \cos (ω t + ϕ)$

$U \sin g E q . (i v), i_{2} = \frac{ω V_{m} c o s (ω t + ϕ)}{(\frac{1}{C} - L ω^{2})}$

$T a k i n g ϕ = 0; i_{2} = \frac{V_{m} \cos (ω t)}{(\frac{1}{ω C} - L ω)} . . . (v)$

Step 4: From Eqs. (i) and (v), we find that

$i_{1} and i_{2}$ are out of phase by

$\frac{π}{2}$ .

$N o w, i_{1} + i_{2} = \frac{V_{m} \sin ω t}{R} + \frac{V_{m} \cos ω t}{(\frac{1}{ω C} - L ω)}$

$P u t \frac{V_{m}}{R} = A = C c o s ϕ a n d \frac{V_{m}}{(\frac{1}{ω C} - L ω)} = B = C \sin ϕ$

$∴ i_{1} + i_{2} = C \cos ϕ \sin ω t + C \sin ϕ \cos ω t$

$= C \sin (ω t + ϕ)$

$w h e r e C = \sqrt{A^{2} + B^{2}}$

$a n d ϕ = \tan^{- 1} (\frac{B}{A})$

$a n d ϕ = \tan^{- 1} [\frac{R}{(\frac{1}{ω C} - L ω)}]$

$H e n c e, i = i_{2} + i_{2} = {[\frac{V_{m}^{2}}{R^{2}} + \frac{V_{m}^{2}}{{(\frac{1}{ω C} - L ω)}^{2}}]}^{1 / 2} \sin (ω t + ϕ)$

$o r \frac{i}{V_{m}} = \frac{1}{Z} = {[\frac{V_{m}^{2}}{R^{2}} + \frac{V_{m}^{2}}{{(\frac{1}{ω C} - L ω)}^{2}}]}^{1 / 2} \sin (ω t + ϕ)$

This is the expression for impedance Z of the circuit.

NEET Information

courses

Company

Botany Questions

Chemistry Questions

Physics Questions

Zoology Questions