1.29 A hollow charged conductor has a tiny hole cut into its surface. Show that the electric field in the hole is (σ2ϵ0ˆnσ2ϵ0^n, where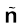 is the unit vector in the outward normal direction, and σ is the surface charge density near the hole.
is the unit vector in the outward normal direction, and σ is the surface charge density near the hole.
Let us consider a conductor with a cavity or a hole. Electric field inside the cavity is zero.
Let E is the electric field just outside the conductor, q is the electric charge, a is the charge density and ε0ε0 is the permittivity of free space.
Charge q = σσ X ds
According to Gauss's law, flux, ∅=E⋅ds=qϵ0∅=E⋅ds=qϵ0
⇒E.ds=σ×dsϵ0∴E=σ2ϵ0ˆn
Therefore, the electric field just outside the conductor is σ2ϵ0ˆn. This field is a superposition of field due to the cavity E and the field due to the rest of the charged conductor E. These fields are equal and opposite inside the conductor and equal in magnitude and direction outside the conductor. ∴E+E′=E
⇒E′=E2=σ2ϵ0ˆn
Hence, the field due to the rest of the conductor is σϵ0ˆn.

© 2025 GoodEd Technologies Pvt. Ltd.
