1.30 Obtain the formula for the electric field due to a long thin wire of uniform linear charge density E without using Gauss’s law. [Hint: Use Coulomb’s law directly and evaluate the necessary integral.]
Take a long thin wire XY (as shown in the following figure) of uniform linear charge density λλ

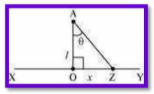
Consider a point A at a perpendicular distance I from the mid-point
O of the wire, as shown in the following figure.
Let E be the electric field at point A due to the wire, XY.
Consider a small length element dx on the wire section with OZ X
Let q be the charge on this piece.
Electric field due to the piece, ∴q=λdx
dE=14πϵ0⋅λdx(AZ)2
However, AZ=√l2+x2
∴ dE =14πϵ0⋅λdx(l2+x2)
The electric field is resolved into two rectangular components. dE cos θ is the perpendicular component and dE sin G is the parallel component. When the whole wire is considered, the component dE sin G is cancelled. Only the perpendicular component dEcos6 affects point A.
Hence, effective electric field at point A due to the element dx is dEj.
In ∆AZO, ∴dE1=14πϵ0⋅λdx⋅cosθ(l2+x2)………………………(1)tanθ=xl⇒x=l.tanθ…………………….(2)
On differentiating equation (2), we obtain
dxdθ=lsec2θ⇒dx=lsec2θdθ……………… (3)
From equation (2), we have
x2+l2=l2tan2θ+l2=l2(tan2θ+1)=l2sec2θ
Putting equations (3) and (4) in equation (1), we obtain
dE1=14πϵ0⋅λsec2θdθ⋅cosθl2sec2θ=14πϵ0⋅λcosθdθl………………………(5)
The wire is so long that 6 tends from −π2 to π2
By integrating equation (5), we obtain the value of field E as,
∫π2−π2dE1=∫π2−π14πϵ0λlcosθdθ⇒E1=14πϵ0λl[sinθ]π2−π2⇒E1=14πϵ0λl×2⇒E1=λ2πϵ0l
Therefore, the electric field due to long wire is λ2πϵ0l.

© 2025 GoodEd Technologies Pvt. Ltd.
