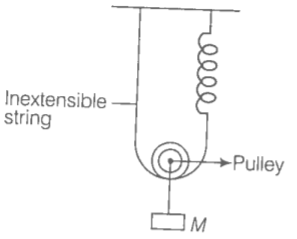
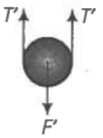
Hint: Use the force method or energy method to find the time period of the system.
Step 1: Find the initial forces on the mass.
For the calculation purpose, in the situation, we will neglect gravity because it is constant throughout and will not effect the net restoring force.
Let in the equilibrium position, the spring has extended by an amount x0.
Now, if the mass is given a further displacement downwards by an amount x, the string and spring both should increase in length by x.
But, string is inextensible, hence the spring alone will contribute the total extension, x+x=2x, to lower the mass down by x from initial equilibrium mean position x0. So, net extension in the spring =2x+x0
Now force on the mass before pulling down, (in the x0 extension case)
F=2T
But T=kx0 [where k is spring constant]
∴ F=2kx0 ...(i)
Step 2: Find the final forces on the mass.
When the mass is lowered down further by x,
F'= 2T'
But new spring length =(2x+x0)
∴ F'=2k(2x+x0) ...(ii)
Restoring force on the system,
Frestoring=-[F'-F]
Using Eqs.(i) and (ii), we get,
Frestroing=-[2k(2x+x0)-2kx0]
=-[2×2kx+2kx0-2kx0]
=-4kx
or Ma=-4kx
where, a=acceleration (As, F=ma)
⇒ a=-(4kM)x
k, M being constant.
∴ a∝-x
Hence, motion is SHM.
Step 3: Find the time period of the mass.
Comparing the above acceleration expression with standard SHM equation, a=-ω2x, we get,
ω2=4kM⇒ω=√4kM
∴ Time period, T=2πω=2π√4kM=2π√M4k