Ncert Exercises & Solutions

A cycle followed by an engine (made of one mole of an ideal gas in a
cylinder with a piston) is shown in the figure. Find heat exchanged by the
engine, with the surroundings for each section of the cycle. [ $C_{V}$ $C_{V}$ = (3/2)R]

(a) AB : constant volume

(b) BC : constant pressure

(d) DA : constant pressure

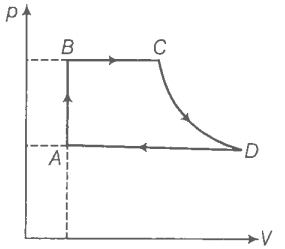
Hint: Use the first law of thermodynamics.

(a) Step 1: Find the heat exchanged by the engine in the process AB.

For process AB,
Volume is constant, hence work done dW = 0
Now, by the first law of thermodynamics,

d Q = d U + d W = d U + 0 = d U

$d Q = d U + d W = d U + 0 = d U$

= n C v d T = n C v (T_{B} - T_{A})

$= n C v d T = n C v (T_{B} - T_{A})$

$= \frac{3}{2} R (T_{B} - T_{A}) (∵ n = 1)$

$= \frac{3}{2} (R T_{B} - R {T_{A}}_{}) = \frac{3}{2} (P_{B} V_{B} - P_{A} V_{A})$

Heat exchanged

$= \frac{3}{2} (P_{B} V_{B} - P_{A} V_{A})$

(b) Step 2: Find the heat exchanged by the engine in the process BC.

For process BC, P=constant

$d Q = d U + d W = \frac{3}{2} R (T_{C} - T_{B}) + P_{B} (V_{C} - V_{B})$

$= \frac{3}{2} (P_{C} V_{C} - P_{B} V_{B}) + P_{B} (V_{C} - V_{B}) = \frac{5}{2} P_{B} (V_{C} - V_{B})$

Heat exchanged

$= \frac{5}{2} P_{B} (V_{C} - V_{A}) (∵ P_{B} = P_{C}$ and

$P_{B} = V_{A})$

Because CD is adiabatic, dQ = the heat exchanged = 0

(d) Step 4: Find the heat exchanged by the engine in process AB.

DA involves compression of gas from

$V_{D}$ to

$V_{A}$ at constant pressure

$P_{A}$ .

$∴$ Heat exchanged can be calculated in a similar way as

$B C$ .

Hence,

$d Q = \frac{5}{2} P_{A} (V_{A} - V_{D})$

NEET Information

courses

Company

Botany Questions

Chemistry Questions

Physics Questions

Zoology Questions