Ncert Exercises & Solutions

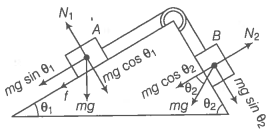
In figure a body \(A\) of mass \(m\) slides on a plane inclined at angle \(\left(\theta_{1}\right)\) to the horizontal and \(\mu\) is the coefficient of friction between \(A\) and the plane. \(A\) is connected by a light string passing over a frictionless pulley to another body \(B,\) also of mass \(m\), sliding on a frictionless plane inclined at an angle \(\left(\theta_{2}\right)\) to the horizontal.

(a)	A will never move up the plane
(b)	A will just start moving up the plane when \(\mu = \dfrac{{\sin} \left(\theta\right)_{2} - {\sin} \left(\theta\right)_{1}}{{\cos} \left(\theta\right)_{1}}\)
(c)	For \(A\) to move up the plane, \(\left(\theta\right)_{2}\) must always be greater than \(\left(\theta\right)_{1}\)
(d)	\(B\) will always slide down with a constant speed

Which of the following statement/s is/are true?

1.	(b, c)	2.	(c, d)
3.	(a, c)	4.	(a, d)

(b, c) Hint: Apply Newton's second law of motion.

Step 1: Find the coefficient of friction.

Let A moves up the plane frictional force on A will be downward as shown.

When A just starts moving up

\begin{array}{l} mg \sin θ_{1} + f = mg \sin θ_{2} \\ \Rightarrow mg \sin θ_{1} + μmg \cos θ_{1} = mg \sin θ_{2} \\ \Rightarrow μ = \frac{\sin θ_{2} - \sin θ_{1}}{\cos θ_{1}} \end{array}

Step 2: Find the friction force.
When A moves upwards

\begin{array}{r} f = mg \sin θ_{2} - mg \sin θ_{1} > 0 \end{array}

\Rightarrow \sin θ_{2} > \sin θ_{1} \Rightarrow θ_{2} > θ_{1}

NEET Information

courses

Company

Botany Questions

Chemistry Questions

Physics Questions

Zoology Questions