Ncert Exercises & Solutions

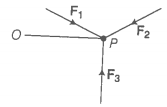
Q. 34 There are three forces $\vec{F_{1}}, \vec{F_{2}} and \vec{F_{3}}$ acting on a body, all acting on a point P on the body. The body is found to move with uniform velocity.

(a) Show that the forces are coplanar.
(b) Show that the torque acting on the body about any point due to these three forces is zero.

Hint: Net force and net torque on the body are zero.

Step 1: As the body moving with uniform speed (velocity) its acceleration,

\vec{a}

= 0

∴

The sum of the forces zero,

\vec{F_{1}} + \vec{F_{2}} + \vec{F_{3}} = 0

(a) Let

\vec{F_{1}}, \vec{F_{2}} and \vec{F_{3}}

be the three forces passing through a point. Let

\vec{F_{1}}

and

\vec{F_{2}}

be in-plane A (one can always draw a plane having two intersecting lines such that the two lines lie on the plane). Then

\vec{F_{1}} + \vec{F_{2}}

must be in-plane A.

Since.

\vec{F_{3}} = - (\vec{F_{1}} + \vec{F_{2}}), \vec{F_{3}}

is also in plane A.

Step 2: Interpret about net torque

(b) Consider the torque of the forces about P Since. all the forces pass through P. the torque is zero. Now, consider torque about another point O. Then torque about O is Torque

= \vec{OP} \times (\vec{F_{1}} + \vec{F_{2}} + \vec{F_{3}})

Since,

\vec{F_{1}} + \vec{F_{2}} + \vec{F_{3}} = 0

torque = 0

NEET Information

courses

Company

Botany Questions

Chemistry Questions

Physics Questions

Zoology Questions