3.23 A three-wheeler starts from rest, accelerates uniformly with 1 m s–2 on a straight road for 10 s, and then moves with uniform velocity. Plot the distance covered by the vehicle during the nth second (n = 1,2,3….) versus n. What do you expect this plot to be during accelerated motion: a straight line or a parabola?
Distance covered by a body in nth second is given by the relation
D=u+a2(2n-1) ...(i)
Where,
u = Initial velocity
a = Acceleration
n = Time = 1, 2, 3, ..... , n
In the given case, u = 0 and a = 1 m/s2
⇒Dn=12(2n-1) ...(ii)
Or, Dn ∝ n ...(iii)Now, substituting different values of n in equation (iii), we get the following table:
|
Dn |
0.5 |
1.5 |
2.5 |
3.5 |
4.5 |
5.5 |
6.5 |
7.5 |
8.5 |
9.5 |
Therefore, the graph between n and Dn will be straight line
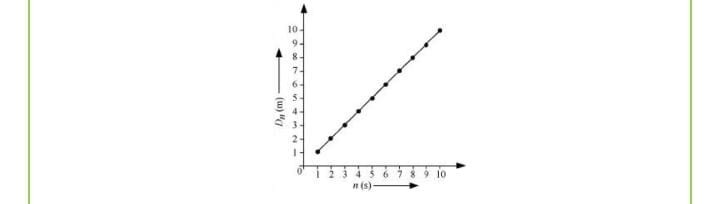
Since the given three-wheeler acquires uniform velocity after 10 s, the line will be parallel to the time-axis after n = 10 s.
3.24 A boy standing on a stationary lift (open from above) throws a ball upwards with the maximum initial speed he can, equal to 49 m s–1. How much time does the ball take to return to his hands? If the lift starts moving up with a uniform speed of 5 m s-1 and the boy again throws the ball up with the maximum speed he can, how long does the ball take to return to his hands?

© 2025 GoodEd Technologies Pvt. Ltd.
