3.25 On a long horizontally moving belt (Fig. 3.26), a child runs to and fro with a speed 9 km h–1 (with respect to the belt) between his father and mother located 50 m apart on the moving belt. The belt moves with a speed of 4 km h–1. For an observer on a stationary platform outside, what is the
(a) speed of the child running in the direction of motion of the belt ?.
(b) speed of the child running opposite to the direction of motion of the belt?
(c) time taken by the child in (a) and (b)?
Which of the answers alter if motion is viewed by one of the parents?
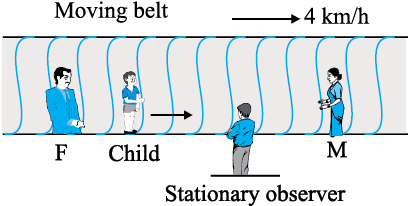
Fig. 3.26

© 2024 GoodEd Technologies Pvt. Ltd.