A particle is projected at an angle θ with horizontal with an initital speed u. When it makes an angle α with horizontal, its speed v is-
1. ucosθ
2. ucosθ ucosα
3. usinθsinα
4. ucosθcosα
A particle is moving along the path y = x2 from x = 0 m to x = 2 m. Then the distance traveled by the particle is:
1. 4 m
2. √20 m
3. >√20 m
4. <√20 m
Six particles situated at the corners of a regular hexagon of side a move at constant speed v. Each particle maintains a direction towards the particle at the next. The time which the particles will take to meet each other is:
1. 2av sec
2. av sec
3. 2a3v sec
4. 3av sec
A body is projected with velocity 20√3 m/s with an angle of projection 60° with horizontal. Calculate velocity on that point where body makes an angle 30° with the horizontal.
1. 20 m/s
2. 20√3 m/s
3. 10√3 m/s
4. 10 m/s
In a uniform circular motion, which of the following quantity is not constant
1. Angular momentum
2. Speed
3. Kinetic energy
4. Momentum
A particle is moving with veocity →v=k(yˆi+xˆj); where k is constant. The general equation for the path is:
1. y=x2+constant
2. y2=x2+constant
3. y=x+constant
4. xy=constant
A particle is projected with a velocity u making an angle θ with the horizontal. At any instant, its velocity v is at right angles to its initial velocity u; then v is:
1. ucosθ
2. utanθ
3. ucotθ
4. usecθ
A projectile is given an initial velocity of ˆi+2ˆj. The cartesian equation of its path is (g = 10 ms-2)
1. y=2x-5x2
2. y=x-5x2
3. 4y=2x-5x2
4. y=2x-25x2
A ship A is moving westwards with a speed of 10 km h-1 and a ship B, 100 km south of A is moving northwards with a speed of 10 km h-1. The time after which the distance between them becomes the shortest, is:
1. 5 hr
2. 5√2 hr
3. 10√2 hr
4. 0 hr
Time taken by the projectile to reach from A to B is t. Then the distance AB is equal to :
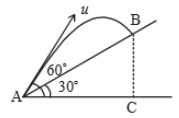

1. ut√3
2. √3ut2
3. √3ut
4. 2ut






